Kurva Matematika kayak Love gitu...!!!

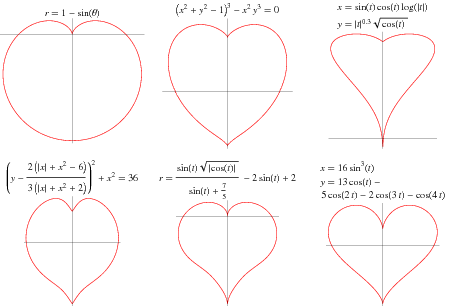
There are a number of mathematical curves that produced heart shapes, sine of which are illustrated above. The first curve is a rotated cardioid (whose name means "heart-shaped") given by the polar equation

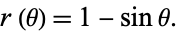
The second is obtained by taking the y=0 cross section of the heart surface and relabeling the z-coordinates as y, giving the order-6 algebraic equation

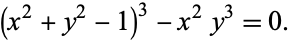
The third curve is given by the parametric equations

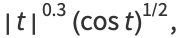
where 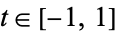(H. Dascanio, pers. comm., June 21, 2003). The fourth curve is given by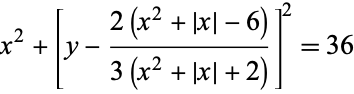

(P. Kuriscak, pers. comm., Feb. 12, 2006). Each half of this heart curve is a portion of an algebraic curve of order 6. And the fifth curve is the polar curve

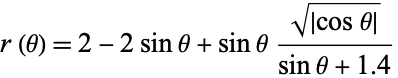
due to an anonymous source and obtained from the log files of Wolfram|Alpha in early February 2010.

Each half of this heart curve is a portion of an algebraic curve of order 12, so the entire curve is a portion of an algebraic curve of order 24.
The areas of these hearts are
A_1 = 3.661972725...
A_2 = 3/2pi
A_3 = 0.237153845...
A_4 = 36pi
A_5 = 12.52...,

where A_5 can be given in closed form as a complicated combination of hypergeometric functions, inverse tangents, and gamma functions.

The Bonne projection is a map projection that maps the surface of a sphere onto a heart-shaped region as illustrated above.
sumber : http://mathworld.wolfram.com/HeartCurve.html